Three Pendulum Rotary Harmonograph Math
When my elder brother and I were kids back in the late 1970’s, our hacker Dad showed us this 1960-61 catalog of the with an interesting graphic design on the cover. He told us to do a thought experiment, asking us to figure out how it would be possible to have a machine that would draw the design on that catalog cover.Incorrectly, our first thought was that the design was created with a. A spirograph has two main parts: a large ring with gear teeth on the inside and outside circumferences and a set of smaller, toothed wheels with holes in them for inserting a drawing instrument — usually a ball point pen. You hold the big ring, insert the pen in the smaller wheel, and then mesh and rotate the smaller wheel around the big ring. But spirographs can’t be used to draw irregular, asymmetrical figures.
You could always recreate a design. Because of the nature of gears, none of them were unique, one off, designs.A spirograph set like this cannot make the image above : Multicherry CC-BY-SA 3.0We figured adding some lever arms, and additional geared wheels (compound gears) could achieve the desired result. It turns out that such a machine is called a. But even with this kind of machine, it was possible to replicate a design as often as required. You would fix the gears and levers and draw a design. If the settings are not disturbed, you can make another copy. Here’s a video of.The eventual answer for making such designs was to use a contraption called as the.
The harmonograph is unique in the sense that while you can make similar looking designs, it would be practically impossible to exactly replicate them — no two will be exactly the same. This thought experiment eventually led to my brother building his own harmonograph. This was way back when the only internet we had was the Library, which was all the way across town and not convenient to pop in on a whim and fancy. This limited our access to information about the device, but eventually, after a couple of months, the project was complete.Getting the Swing of ThingsIt quickly dawned on us that using gears wasn’t the solution to this problem. A pendulum clock gave us some pointers about a plausible solution.
Much later we learned that the earliest ‘lateral’ harmonographs consisted of single axis pendulums. One moved the pen laterally along the drawing platform, while the other moved the drawing platform perpendicular to the pen. Their limited movement resulted in Lissajous curves.
If the pendulums were instead allowed more degrees of movement by fixing them to gimbals, more complex designs were possible. Harmonograph seen at BAMF 2016 But getting from theory to practice isn’t always easy. We could tie string to a pen and weigh it down with some weights, attach it to a hook, and swing it around. That might work, but we would need a spherical (concave) surface for the pen to make constant contact. Here’s an example that shows.
Or we could use a free floating or spring damped pen which is able to move freely in the vertical axis to ensure that it is in contact with the paper while the pendulum swings around. Jonathan Lansey describes the construction of such a single pendulum, along with a detailed mathematical analysis. Our PrototypeAfter some more time with our thinking caps on, we finally had a good idea of how to build our device — a dual pendulum design, with one pendulum moving the pen and the other one moving the drawing platform. We could attach a rigid pendulum to a gimbal, fix a hinged lever on top of it and a pen at the end of the lever. Move the pendulum and the pen will move too, but will stay in constant contact with the paper because of the hinged lever. All we had in terms of plans was hand drawn sketches. We tried using material we had lying around, so there were a lot of changes going from sketches to finished parts.

It helped that we had our own workshop with all the tools and machines needed to build it.Our final design consisted of a welded steel frame that could be cantilevered to the edge of a table, so it wasn’t a self-standing design. The frame held two gimbals, each having a pendulum. One carried the pen lever with its counter weight, while the other was used to move the drawing platform. Adjusting the pendulum height changed the time constant, and adjusting the pen lever counter weight controlled how firmly the pen pushed on the paper. More weight caused friction, slowing down the pendulum faster. Less weight and the pen lines became whisker thin. The new version, seen in the video below, was built a couple of years back and is similar to the one we built in the ‘70’s, except it is completely self standing and portable.
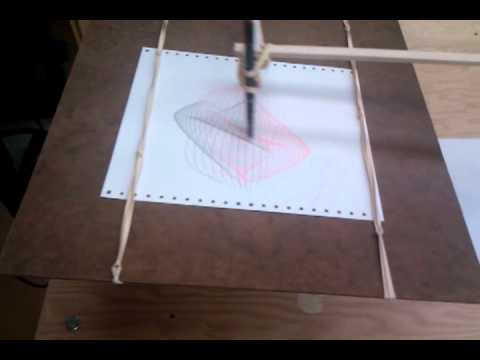
Harmonograph Design ImprovementsIt’s possible to add additional degrees of movement to create even more stunning art. Instead of moving the pen via a single pendulum, you could use two pendulums and get the pen to move in an even more complex motion. Karl Sims describes the construction of such a which is simple to fabricate. Or you can attach two, or even three, pens and create multi-colored designs.In 2017, with the comfort of having instant access to practically any kind of information, it’s easy to learn a lot more about the harmonograph than we could back in the ’70’s. For example, features a nice one page overview of the harmonograph, giving some insight into its past history. And Anthony Ashton’s book is considered a definitive reference on this subject.

Do a cursory search for harmonograph images in any search engine and you will have hundreds of beautiful pictures to admire.We featured a, built using Karl Sims’s plans a long while ago and were wondering if any of you hackers out there have built another one? Let us know.Posted in, Tagged, Post navigation. I seem to recall when I was much younger the science centre in Canberra (Questacon)had a large harmonograph it was basically a large heavey platform suspended in each corner by wire the pen was fixed on an arm which only moved up and down. The paper sat on the platform and moved with the platform under the pen.I it’s early days questacon was a hackers delight all the exhibits were built with the minimum of fuss and polish and maintained and displayed by what I believe was a team of dedicated volunteers. When it moved from the old school to a prominent new building the level of polish on the constructions went up along with the comecialisation. It’s still a great place to visit.
Pfft nothing new there, for a more original original hack try a pan of water (even mercury or any liquid metal alloy), a laser pointer, multiple speakers or vibration sources and some photosensitive whatever, perhaps even coated PCB board of you want a robust artefact that you can gold plate. It doesn’t have to be big either as another laser can then be used to project a larger reflection off it for display. Or you can just have the laser path (UV) go through UV curing resin for a 3D version of the same thing, that can even act as a lens for another laser to create a frozen ripple reflection effect. Late 1960 – 1970’s Hewlett-Packard had devices called “plotters” that connected to their range of desk-top computers.
These were primarily for cad-type applications and had the ability to produce multi-colour drawings under the control of the computer. One of the demonstration programs that drew the crowds at exhibitions was a program they called “The Birthday Plot”. This called for the input of a six-figure number (the birthday) into the program and would produce a multi-colour, drawn image on the plotter, as per the harmonograph. As I recall, the inventor of the Spirograph, Denys Fisher, purchased similar equipment from Hewlett-Pack to further improve and “re-invent” the Spirograph.
Topics: Connecting nature, music and number, Pythagorean theorem, music from math curves, sin and cos functions, the Python math library, visualizing oscillations, the harmonograph, sonifying oscillations, Kepler’s harmony of the world revisited.In the previous chapters, we studied essential building blocks of music and computer science. We now know enough about music and programming to return to the themes introduced in. In this chapter, we will deepen our exploration into the connections between music, number, and nature, and introduce you to ideas that will hopefully inspire and guide you in your own personal journey into music and programming. More information is provided in the.Here is code from this chapter:.Making music from math curvesA simple mathematical function is the sine. It describes a smooth repetitive oscillation (i.e., a wave), as shown below.
A lateral harmonographThe code sample below simulates a lateral harmonograph. We may adjust:. Length of pendula — this affects frequency of oscillation. By combining different frequency ratios (e.g., 2:3), we get different shapes (as shown above).
Phase of pendula, relative to one another. This can be same, or reverse.Here is the code:# harmonographLateral.py## Demonstrates how to create a lateral (2-pendulum) harmonograph# in Python.## See Ashton, A. A rotary harmonographThe code sample below simulates a rotary harmonograph.
We may adjust:. Length of pendula — this affects frequency of oscillation. By combining different frequency ratios (e.g., 2:3), we get different shapes (as shown above). Phase of pendula, relative to one another. This can be same, or reverse.This program can also dampen oscillations (via friction). To do so, uncomment the last two statements. This introduces more interesting shapes.Here is the code:# harmonographRotary.py## Demonstrates how to create a rotary (3-pendulum) harmonograph# in Python.## Here, the position of the pen is determined by two pendula,# and is modeled by either (sin, sin) or (cos, sin).# The third pendulum has its own sin and cos to model the second# circle.## See Ashton, A.
Three Pendulum Rotary Harmonograph Math Worksheets
(Left) Lateral harmonograph – 5.01: 4 ratio, same phase, 6 times. (Right) Rotary harmonograph – 9.13: 7 ratio, same phase, 6 times.Certain ratios result in paths that will never converge (i.e., never re-trace the same path).NOTE: The faster a ratio begins to retrace the same path, the more harmonious (consonant) it sounds to our ear.
See Legname’s Theory on Density of Intervals.Kepler’s Harmony of the World, No. 2Here is another sonification of the planets, influenced by the harmonograph above (also see ).This code sample sonifies planetary velocities. It uses sines and cosines to simulate movement (sound spatialization).Here is the code:# harmonicesMundiRevisisted.py## Sonify mean planetary velocities in the solar system.#from music import.from math import.from random import.# Create a list of planet mean orbital velocities# Mercury, Venus, Earth, Mars, Ceres, Jupiter, Saturn, Uranus,# Neptune.